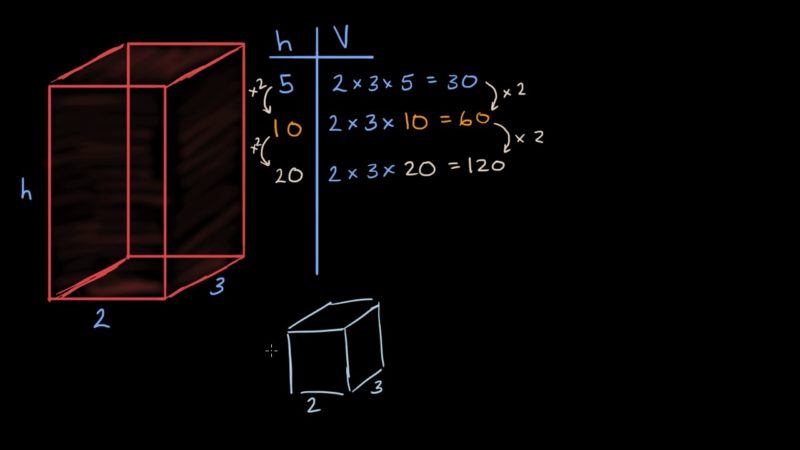
The rectangle is one of the geometric shapes which is considered a two-dimensional shape. A prism is defined as a polyhedron that has identical or similar bases. It is classified into various types based on the sizes of its dimensions. The volume of the rectangular prism is regarded as the total area which is occupied by the prism by its three dimensions. Mathematically, the volume of the rectangular prism is l * b * h where l is the length, b is the breadth and h is the height of the rectangular prism. We shall cover various interesting topics such as the volume of a triangular prism, some examples based on it, and many more.
Some Examples Based on the Volume of Rectangular Prism
To recall, the mathematical formula to calculate the volume of a rectangular prism is l * b * h where l is the length, b is the breadth and h is the height of the rectangular prism. To excel in any subject, one must solve various types of examples related to it. Let us solve some examples based on the volume of the rectangular prism. Some of them are listed below:
Example 1: Find the volume of a rectangular prism if the length, height, and breadth of the prism is 3 cm, 4 cm, and 5 cm respectively.
Solution: According to the question,
Length of the rectangular prism = 3 cm
The breadth of the rectangular prism = 5 cm
Height of the rectangular prism = 4 cm
Using the formula of the volume of rectangular prism = l * b * h.
3 * 4 * 5 = 60 cm cubic units.
Thus, the volume of the rectangular prism for the given values is equivalent to 60 cm cubic units.
Example 2: Find the volume of a rectangular prism if the length, height, and breadth of the prism is 4 cm, 6 cm, and 8 cm respectively.
Solution: According to the question,
Length of the rectangular prism = 4 cm
The breadth of the rectangular prism = 8 cm
Height of the rectangular prism = 6 cm
Using the formula of the volume of rectangular prism = l * b * h.
4 * 8 * 6 = 192 cm cubic units.
Thus, the volume of the rectangular prism for the given values is equivalent to 192 cm cubic units.
Volume of the Triangular Prism
As mentioned above, the prism is classified into various types based on the sizes of its dimensions. One of them is the triangular prism. The volume of the triangular prism can be defined as the total area which is occupied by the prism by its three dimensions. The mathematical formula given to find the volume of a triangular prism is the base area of the triangular prism multiplied by the length of the prism. The base area of the prism is calculated by multiplying the base of the triangle and the height of the triangle. We shall cover some examples based on the volume of the triangular prism in the coming sections.
Some Examples Based on the Volume of Triangular Prism
The mathematical formula given to find the volume of a triangular prism is the base area of the triangular prism multiplied by the length of the prism. Some related examples are discussed below.
Example 1: Find the volume of the triangular prism if the base of the triangle is 7 cm, height is 5 cm and the length of the prism is 10 cm.
Solution: Given that,
The base of the triangle = 7 cm
Height of the triangle = 5 cm
Hence, the base area = 1/2 * b *h
1/2 * 7 cm * 5 cm = 35/2 or 17.5 cm square units.
Now, the volume of the triangular prism = length of the prism * base area.
35/2 * 10 = 175 cm cubic units.
Therefore, the volume of the triangular prism for the given length, height, and base is 175 cm cubic units.
Visit Cuemath to study more about these concepts in a detailed, fun, and interactive manner. Go and book a free session now.